Leading the world in
energy absorption

| Kinetic energy to be absorbed | 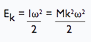 |
| Energy due to drive force | 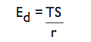 |
| Total energy to be absorbed | ET = Ek + Ed |
| Maximum impact force |  |
| Design mass for buffer | 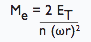 |
To avoid confusing conventions within calculations always use SI units in formulae then convert to more appropriate units if required.
| Notation | Quantity | SI Unit |
| M | Mass of body | kg |
| Me | Buffer design mass | kg |
| S | Buffer stroke | m |
| K | Radius of gyration | m |
| Ek | Kinetic energy | J |
| Ed | Energy due to drive force | J |
| Et | Total energy | J |
| ω | Angular velocity | rad/s |
| I | Moment of inertiaa | kg.m2 |
| T | Torque | Nm |
| F | Impact force | N |
| N | Number of buffers in parallel | – |
| ξ | Efficiency | - |
Eg. Consider a swing bridge, having a moment of inertia (I) of 7500000 kgm2, buffer arm radius (r) 8m, angular velocity (ω) of 0.174 rad/sec and a driving torque (T) of 1500000 Nm. Using 2 buffers.
To find the energy to be absorbed:
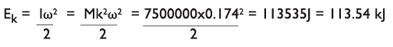
Let us select a Type 4 with 114mm stroke:

Total energy to be absorbed:
Therefore ET = Ek + Ed = 113535 + 21375 = 134910 J = 134.91 kJ
To find the maximum impact force:
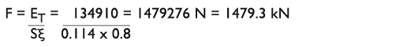
§To find the equivalent mass for metering pin selection:
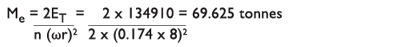
Therefore select metering pin code 08 for masses up to 80000kg (80 tonnes).